Flat Earth Failures: Perspective and Vanishing Point
I often see Flat Earthers appealing to 'perspective' and 'vanishing point' to explain why so many of our observations appear as if the Earth is a globe. However, they seem to be unable to explain anything in detail about these phenomena and rather just use these two terms as excuses.
So I wanted to take a detailed look at what these two terms really mean.
First of all 'vanishing point' is an imaginary point at which parallel lines would appear to converge if extended to infinity - imaginary and appear being key words here. There is zero evidence this is an actual point or distance away at which anything magically 'vanishes'. So that's just inane.
Perspective can be summed up as the following relationship:
This simply says that the apparent size of an object is equal to the height divided by the distance.
That is the essence of perspective. We will revisit this in more detail below after we review a few of the problems that any Flat Earth claim faces.
Why does the sun set at the horizon when it supposedly remains above the plane of the Earth?
There is no distance at which we should be unable to see the Sun if it remained above us.
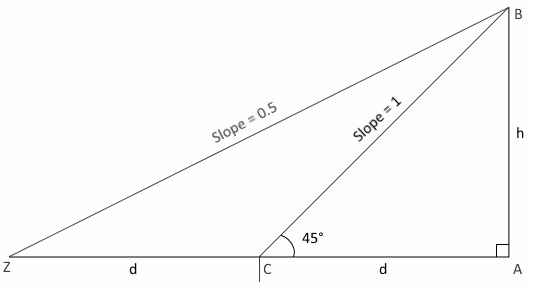
Consider the Equinox when the sun is directly above the equator. At 45°N Latitude the sun appears 45° above the horizon (slope equals 1). If we double that distance by moving to the North Pole then the angle to the Sun should be a slope of 0.5 (26.565° above the horizon) on a Flat Earth -- but it isn't -- it's all the way down on the horizon, exactly where a distant Sun should appear if we're on a globe.
[see more detailed analysis]
How does the sun maintain the same angular size while getting massively further away?
This is the opposite of perspective. I've seen some Flat Earthers appeal to some magical version of 'atmospheric lensing' which they also cannot explain. Why doesn't this effect happen to mountains and buildings? What mechanism causes this so reliably but is excluded from all other observations?
So I wanted to take a detailed look at what these two terms really mean.
First of all 'vanishing point' is an imaginary point at which parallel lines would appear to converge if extended to infinity - imaginary and appear being key words here. There is zero evidence this is an actual point or distance away at which anything magically 'vanishes'. So that's just inane.
Perspective can be summed up as the following relationship:
 |
| Figure 1 |
This simply says that the apparent size of an object is equal to the height divided by the distance.
That is the essence of perspective. We will revisit this in more detail below after we review a few of the problems that any Flat Earth claim faces.
Why does the sun set at the horizon when it supposedly remains above the plane of the Earth?
There is no distance at which we should be unable to see the Sun if it remained above us.
Consider the Equinox when the sun is directly above the equator. At 45°N Latitude the sun appears 45° above the horizon (slope equals 1). If we double that distance by moving to the North Pole then the angle to the Sun should be a slope of 0.5 (26.565° above the horizon) on a Flat Earth -- but it isn't -- it's all the way down on the horizon, exactly where a distant Sun should appear if we're on a globe.
 |
| Figure 2 |
[see more detailed analysis]
How does the sun maintain the same angular size while getting massively further away?
This is the opposite of perspective. I've seen some Flat Earthers appeal to some magical version of 'atmospheric lensing' which they also cannot explain. Why doesn't this effect happen to mountains and buildings? What mechanism causes this so reliably but is excluded from all other observations?
They complain there is "no evidence" for 'Gravity' but disingenuously turn around and appeal to utterly unevidenced magic.
Why do the bottoms of mountains and tall buildings disappear behind the curvature?
For example this is Mt. Rainer from about 130 miles away. We can see the top perfectly fine.
How about Chicago, which they will claim is a PERFECTLY undistorted image all the way down to the shore...
But looks NOTHING like the actual skyline, proportions are highly distorted, buildings are flattened... In short, it shows every evidence of extreme refractive effects.
[see more detailed analysis]
Why doesn't zooming in bring back objects that are shown to be more distant than the horizon point?
What Flat Earthers like to do is take extremely poor quality video of boats that ARE NOT actually distant enough to be over the horizon and then zoom in and pretend this proves that zooming in brings back things that were over the horizon. But they ignore the High Quality footage with extremely high optical zoom that clearly demonstrates that zooming in does NOT enlarge any part of the distant city which is clearly distant enough to be over the horizon.
Figure 9
Well, I challenge any Flat Earther to try to explain how their 'perspective' actually works. And I don't mean a bunch of hand waving, I want them to share their mathematical model and show how they demonstrated that their model is more accurate than SIZE/DISTANCE -- which simply cannot hide a building behind flat ground.
So, can you share your mathematical model of 3D perspective and then, using that model, explain how buildings and mountains magically melt into the ground with distance?
Here is how 3D perspective works in reality.
Each 3D coordinate is mapped into a 2D planar view using the following relationship:
3D [x,y,z] -> 2D [x/z, y/z] (with 0,0 being the center of our view)
You'll notice that this is exactly the same as our previous formula where the apparent size equals the height (x for horizontal and y for vertical here) divided by the distance (z).
That's ALL that perspective is. Things get smaller with distance.
We can use this to map a bunch of points out in space to where they would appear in a photograph (or on your retina).
Let's say we have a distant building (simplified to just one vertical line here) that goes from [0, 0, 100] to [0, 50, 100] (so it's 50 y units tall, at 100 z units distant) and some water in front of it that covers [0,0,50] to [0,20,50] (so the water is closer to us at z = 50 units and only 20 y units high).
These coordinates map to:
[0, 1/2] << top of building (50/100)
[0, 2/5] << top of water, which begins to hide the more distant building
[0, 0] << bottom of water AND bottom of building
Because the water was closer it will occlude the more distant building.
So we see water up to 2/5 and then building up to 1/2 in our projected view.
But if that water is FLAT then it's ALWAYS at [0,0,*] -- every Z distance is 0, so it's ALWAYS at [0,0] along that line of sight. So now we get a mapping of:
[0, 1/2] << top of building (50/100) -- we see the ENTIRE building - just smaller because it's more distant
[0, 0] << water AND bottom of building
What if we move that building to be ten times further, at 1000 z units away?
[0, 1/20] << top of building (50/1000) -- we STILL see the ENTIRE building - just equally smaller because it's more distant
[0, 0] << water AND bottom of building
No matter how far away you move that building, every single foot of the entire building is going to be the same angular size from our view. You will never see only the top of the building and have the bottom missing due to "perspective" smashing it into the ground.
So this means that otherwise parallel lines receding from our view get closer to together but never actually converge and, AT NO POINT, would an object that is above some line of sight be hidden by an object BELOW it.
So if your eye is above the ground and you are looking straight out, the ground could NEVER hide part of a building at any distance. That would violate the actual Law of Perspective. If you change the angles then sure, something closer can hide something further away but it has to be IN your line of sight to do so -- it cannot be a plane that lies BELOW your line of sight.
So the Flat Earth appeal to perspective falls flat.
Your turn.
Here is a hilarious look at how one Flat Earther thinks distance will make the Sun shine UNDERNEATH a cloud because the Sun is far away and the Cloud is closer. This is exactly what I was saying above -- they literally think that perspective makes the more distant object magically be underneath a closer object.
| Figure 4 |
Why do the bottoms of mountains and tall buildings disappear behind the curvature?
For example this is Mt. Rainer from about 130 miles away. We can see the top perfectly fine.
 |
| Figure 5 |
But where did the bottom go?
 |
| Figure 6 |
[see more detailed analysis]
 |
| Figure 7 |
But looks NOTHING like the actual skyline, proportions are highly distorted, buildings are flattened... In short, it shows every evidence of extreme refractive effects.
 |
| Figure 8 |
[see more detailed analysis]
Why doesn't zooming in bring back objects that are shown to be more distant than the horizon point?
What Flat Earthers like to do is take extremely poor quality video of boats that ARE NOT actually distant enough to be over the horizon and then zoom in and pretend this proves that zooming in brings back things that were over the horizon. But they ignore the High Quality footage with extremely high optical zoom that clearly demonstrates that zooming in does NOT enlarge any part of the distant city which is clearly distant enough to be over the horizon.
Well, I challenge any Flat Earther to try to explain how their 'perspective' actually works. And I don't mean a bunch of hand waving, I want them to share their mathematical model and show how they demonstrated that their model is more accurate than SIZE/DISTANCE -- which simply cannot hide a building behind flat ground.
So, can you share your mathematical model of 3D perspective and then, using that model, explain how buildings and mountains magically melt into the ground with distance?
How 3D Perspective Works
Here is how 3D perspective works in reality.
Each 3D coordinate is mapped into a 2D planar view using the following relationship:
3D [x,y,z] -> 2D [x/z, y/z] (with 0,0 being the center of our view)
 |
| Figure 10 |
You'll notice that this is exactly the same as our previous formula where the apparent size equals the height (x for horizontal and y for vertical here) divided by the distance (z).
That's ALL that perspective is. Things get smaller with distance.
Example
We can use this to map a bunch of points out in space to where they would appear in a photograph (or on your retina).
Let's say we have a distant building (simplified to just one vertical line here) that goes from [0, 0, 100] to [0, 50, 100] (so it's 50 y units tall, at 100 z units distant) and some water in front of it that covers [0,0,50] to [0,20,50] (so the water is closer to us at z = 50 units and only 20 y units high).
These coordinates map to:
[0, 1/2] << top of building (50/100)
[0, 2/5] << top of water, which begins to hide the more distant building
[0, 0] << bottom of water AND bottom of building
Because the water was closer it will occlude the more distant building.
So we see water up to 2/5 and then building up to 1/2 in our projected view.
But if that water is FLAT then it's ALWAYS at [0,0,*] -- every Z distance is 0, so it's ALWAYS at [0,0] along that line of sight. So now we get a mapping of:
[0, 1/2] << top of building (50/100) -- we see the ENTIRE building - just smaller because it's more distant
[0, 0] << water AND bottom of building
What if we move that building to be ten times further, at 1000 z units away?
[0, 1/20] << top of building (50/1000) -- we STILL see the ENTIRE building - just equally smaller because it's more distant
[0, 0] << water AND bottom of building
No matter how far away you move that building, every single foot of the entire building is going to be the same angular size from our view. You will never see only the top of the building and have the bottom missing due to "perspective" smashing it into the ground.
So this means that otherwise parallel lines receding from our view get closer to together but never actually converge and, AT NO POINT, would an object that is above some line of sight be hidden by an object BELOW it.
So if your eye is above the ground and you are looking straight out, the ground could NEVER hide part of a building at any distance. That would violate the actual Law of Perspective. If you change the angles then sure, something closer can hide something further away but it has to be IN your line of sight to do so -- it cannot be a plane that lies BELOW your line of sight.
 |
| Figure 11 |
Your turn.
Here is a hilarious look at how one Flat Earther thinks distance will make the Sun shine UNDERNEATH a cloud because the Sun is far away and the Cloud is closer. This is exactly what I was saying above -- they literally think that perspective makes the more distant object magically be underneath a closer object.


Comments
Post a Comment